Solution des exercices : Travail et puissance mécaniques - 1er s
Classe:
Première
Exercice 1
Un système constitué de deux blocs reliés par un fil AB de masse négligeable est tiré avec une force constante F, d'intensité 300N, sur un plan horizontal rugueux.
On donne α=60∘
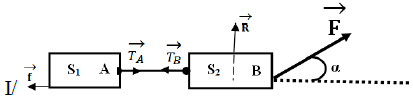
1. Calcul du travail de la force →F lorsque le système s'est déplacé de CD=20m. On a :
WCD(→F)=→F⋅→CD=F×CDcosα=300×20cos60∘=3.0⋅103
Ainsi, WCD(→F)=3.0⋅103J
2. La vitesse étant constante, la tension du fil horizontal AB qui relie les deux blocs est alors constante et égale à 120N.
Calculons le travail au cours du trajet de la tension du fil appliqué au bloc S2 et le travail de la tension du fil appliqué au bloc S1. Soit :
WCD(→TB)=→TB⋅→CD=−TB×CD=−120×20=−2.4⋅103
Donc, WCD(→TB)=−2.4⋅103J
Par ailleurs, comme →TB+→TA=→0 alors, TA=TB
Par suite,
WCD(→TA)=→TA⋅→CD=TA×CD=TB×CD=−WCD(→TB)=2.4⋅103
D'où, WCD(→TA)=2.4⋅103J
Calcul du travail total des forces de frottement exercées par le plan sur S1 et S2.
La vitesse est constante, le principe de l'inertie appliqué au système constitué du bloc S1 et du bloc S2 s'écrit :
→F+→f=→0⇒Fcosα−f=0⇒f=Fcosα
Par suite :
WCD(→f)=→f⋅→CD=−f×CD=−F×CDcosα=−3.0⋅103
D'où, WCD(→f)=−3.0⋅103J
3. On envisage maintenant le cas où la vitesse n'est plus constante ; la tension du fil varie au cours du mouvement.
Dans ce contexte, les travaux de la tension →TB appliquée en B et de la tension →TA appliquée en A dépendent du chemin suivi.
Exercice 2
Alpha tire, à vitesse constante, une luge de masse m=6.00kg sur un sol horizontal ; la distance parcourue est d=AB=100m.
La force →F exercée sur la luge par l'intermédiaire de la corde est constante sur la distance d ; la corde fait un angle α=30.0∘ avec le sol.
On suppose que la valeur f des forces de frottements vaut le cinquième du poids P de la luge.
1. Inventaire des forces qui s'exercent sur le système {Alpha+luge}.
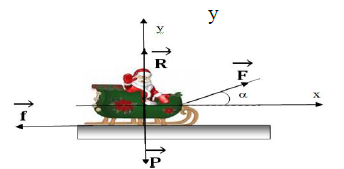
2. Calcul de la valeur de la force de traction qu'exerce Alpha sur sa luge.
Système : {Alpha+luge}
Bilan des forces appliquées : →F : →P : →R : →f
Le système évolue à vitesse constante, le principe de l'inertie s'écrit :
→F+→P+→R+→f=→0
En projetant la relation vectorielle suivant l'axe des x, il vient :
Fcosα+0+0−f=0⇒Fcosα=f⇒F=fcosαor f=P5=mg5⇒F=mg5cosα⇒F=6.00×9.815cos30.0∘⇒F=13.6N
D'où, F=13.6N
3. Calcul du travail de chacune des forces le long du trajet.
WAB(→F)=→F⋅→AB=Fdcosα=13.6×100cos30.0∘=11.8⋅102
D'où, WAB(→F)=11.8⋅102J
WAB(→P)=→P⋅→AB=0
Or, →P⊥→AB donc, →P⋅→AB=0
D'où, WAB(→P)=0J
WAB(→f)=→f⋅→AB=−fd=−P5d=−mg5d=−6.00×9.815×100=−11.8⋅102J
Ainsi, WAB(→f)=−11.8⋅102J
WAB(→R)=→R⋅→AB=0J car →R⊥→AB
Alpha aborde maintenant la piste de luge (de longueur l=100m) qui forme un plan incliné d'un angle β=15.0∘ avec l'horizontale.
Elle tire toujours rectilignement et à vitesse constante ; l'angle entre la corde et la pente est toujours de 30.0∘.
On suppose que la force de frottements garde la même valeur f que précédemment.
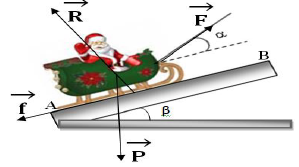
4. Le travail de la somme des forces est nul.
En effet,
→v=→cte⇒→F+→P+→R+→f=→0⇒→F⋅→AB+→P⋅→AB+→R⋅→AB=→0⋅→AB⇒WAB(→F)+WAB(→P)+WAB(→R)+WAB(→f)=0
5. Donnons l'expression du travail de chacune des forces s'exerçant sur la luge.
WAB(→F)=→F⋅→AB=Flcosβ
WAB(→P)=→P⋅→AB=−Plsinβ
WAB(→R)=→R⋅→AB=0 car →R⊥→AB
WAB(→f)=→f⋅→AB=−fl
6. Comparons les valeurs de la force de traction sur la partie plane et sur la pente.
Sur la partie pente :
WAB(→F)=→F⋅→AB=Flcosβ=13.6×100×cos15.0∘=13.1⋅102
Donc, sur la pente : WAB(→F)=13.1⋅102J
Sur la partie rectiligne : WAB(→F)=11.8⋅102J
Sur la partie pente, Alpha fournit plus d'efforts.
C'est pourquoi WAB(→F)=13.1⋅102J>WAB(→F)=11.8⋅102J
7. Le déplacement est effectué en 2.0min.
Calculons la puissance moyenne du travail du poids.
On a :
Pm(→P)=WAB(→P)Δt=−PlsinβΔt=−6.00×9.81×100×sin15.02.0×60=−12.7
D'où, Pm(→P)=−12.7W
Exercice 3
Un chariot de masse M=20Kg tiré le long d'une piste horizontale AB de longueur L=4m par une force →F inclinée d'un angle α=60∘ par rapport au déplacement et de valeurs F=120N (voir fig).
On néglige tous les frottements.
Le long du trajet AB, le chariot est tiré avec une vitesse constante =1m⋅s−1.
1. Expression et calcul du travail effectué par →F le long du trajet AB.
On a :
WAB(→F)=→F⋅→AB=Flcosα=120×4×cos60∘=240
D'où, WAB(→F)=240J
2. Expression et calcul de la puissance moyenne développée par cette force
On a :
Pm(→F)=→F→v=Fv=120×1=−120
Ainsi, Pm(→F)=−120W
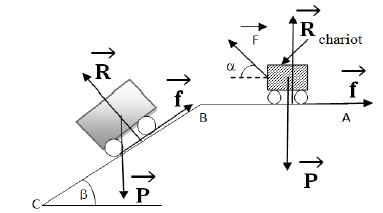
3. En arrivant au point B, on supprime la force motrice →F et le chariot aborde une piste BC de longueur L′ incliné par rapport à l'horizontale passant par C d'un angle β=30∘.
Le long du trajet BC, le chariot est soumis à des forces de frottement équivalente à une force →f constamment opposé au déplacement et de valeur f=30N.
La différence d'altitude entre les points B et C est h=2m.
a) Expression et calcul du travail du poids →P du chariot.
Soit :
WBC(→P)=→P⋅→BC=Mgh=20×10×2=4⋅102
Alors, WAB(→P)=4⋅102J
b) Expression et calcul du travail de la force de frottement.
On a :
WBC(→f)=→f⋅→BC=−Fl=−fhsinβ=−30×2sin30∘=4−120
D'où, WAB(→f)=4−120J
c) Expression et calcul du travail de la réaction →R du plan
Soit : WAB(→R)=→R⋅→AB=0 car →R⊥→AB
Exercice 4
Une barre est maintenue horizontale par l'intermédiaire d'un fil métallique et un fil de coton fixés en son milieu.
Les deux fils sont verticaux, le fil métallique est au-dessus de la barre, le fil de coton en dessous.
Le fil métallique a une constante de torsion C=4.0⋅10−2N⋅m⋅rad−1.
Le fil de coton exerce un couple négligeable.
1. Schéma du dispositif.
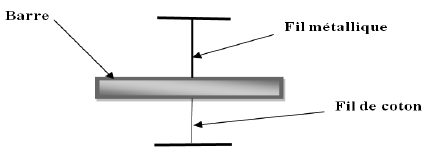
2. Calcul du travail du couple de torsion dans les situations suivantes :
2.1. La barre écartée de 90∘ par rapport à sa position d'équilibre. Alors, on a :
WC=−C(gf−gi)=−4.0⋅10−2(π2−0)=−6.28⋅10−2
Donc, WC=−6.28⋅10−2J
2.2 Lorsque la barre passe de la position précédente à la position où elle fait un angle de 45∘ par rapport à sa position d'équilibre.
Soit alors :
WC=−C(gf−gi)=−4.0⋅10−2(π4−π2)=3.14⋅10−2
D'où, WC=3.14⋅10−2J
2.3 Lorsque la barre passe de cette dernière position à la position où elle est écartée d'un angle de 30∘ de l'autre côté de sa position d'équilibre.
WC=−C(gf−gi)=−4.0⋅10−2(−π6−π4)=5.24⋅10−2
Ainsi, WC=5.24⋅10−2J
Exercice 5
Pour hisser, à vitesse constante, un corps sur une plateforme on utilise un treuil entraîné par un moteur (fig 1).
La masse du corps M=1000kg, la hauteur h=2m et les frottements créent une force f de direction opposée au déplacement.
La force motrice F=10000N pour un angle α=30∘.
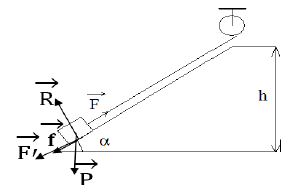
1. Calculons la force résistante (→F′) présentée par le poids : F′=P⋅sinα (sens opposé au déplacement) et la force de frottement.
Soit :
→F′=P⋅sinα=Mgsinα=1000×10×sin30∘=5.0⋅103
Donc, F′=5.0⋅103N
Le corps se déplace à vitesse constante, le principe de l'inertie s'écrit :
→F+→P+→R+→f=→0
En projetant suivant le sens de la force motrice, il vient :
F−F′+0−f=0⇒f=F−F′⇒f=1000−5.0⋅105⇒f=5.0⋅105
D'où, f=5.0⋅105N
2. Calculons le travail de la force →F et la puissance correspondante si la masse se déplace à 0.2m/s.
Soit :
W(→F)=Fhsinα=10000×2sin30∘=4.0⋅104
Alors, W(→F)=4.0⋅104J
Pour la puissance, on obtient :
P(→F)=→F⋅→v=Fv=10000×0.2=2.0⋅103
Ainsi, P(→F)=2.0⋅103W
Le treuil ayant un diamètre de 20cm et un rendement de 0.85
3. Calculons :
− la puissance mécanique du moteur nécessaire
On a :
P(→F)PM=0.85⇒PM=P(→F)0.85⇒PM=2.0⋅1030.85⇒PM=2.4⋅103
Donc, PM=2.4⋅103W
− la vitesse angulaire de rotation,
Soit :
ω=vr=vd2=2vd=2×0.220⋅10−2=20
Ainsi, ω=20rad⋅s−1
− la fréquence de rotation (tr/min)
On a :
ω=2πN⇒N=ω2π⇒N=202π⇒N=3Hz
En convertissant, on obtient :
N=3Hz=3×60=180tr/mn
D'où, N=180tr/mn
− le moment du couple moteur
Soit :
PM=MCω⇒MC=PMω⇒MC=2.4⋅10320⇒MC=1.2⋅102Nm
Par suite, MC=1.2⋅102Nm
Exercice 6
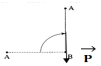
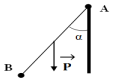
Une tige de cuivre supporte deux boules de fer (m1=m2=m).
L'ensemble est mobile sans frottement autour d'un axe horizontal Δ, qui est perpendiculaire en O, au plan de la figure.
Le centre d'inertie de la barre de masse (M) est à la distance OG=a de l'axe.
Un aimant attire la boule de fer en A1, avec une force horizontale F1 ; un deuxième aimant attire la boule de fer A2 avec une force F2.
On pose OA1=ℓ1 et OA2=ℓ2
1. La tige fait un angle α avec la verticale.
a) Représentons les forces extérieures appliquées sur le système (tige + boules)
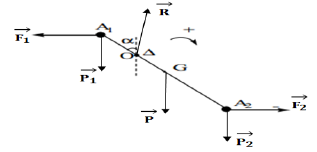
b) Exprimons littéralement les moments de ces forces par rapport à l'axe Δ en fonction des données.
On a :
MΔ(→F1)=−F1OA1cosα=−F1l1cosα
Soit : MΔ(→F1)=−F1l1cosα
On a :
MΔ(→F2)=−F2OA2cosα=−F2l2cosα
Soit alors : MΔ(→F2)=−F2l1cosα
On a :
MΔ(→P)=POGcosα=Mgacosα
Ainsi, MΔ(→P)=Mgacosα
Soit :
MΔ(→P1)=−P1OA1sinα=−mgl1sinα
Donc, MΔ(→P1)=−mgl1sinα
On a :
MΔ(→P2)=P2OA2sinα=mgl2sinα
D'où, MΔ(→P2)=mgl2sinα
Enfin, MΔ(→R)=0
2. Pour la valeur de =α=20∘, l'ensemble est en équilibre.
Déterminons alors l'intensité commune F des forces →F1 et →F2
Soit :
MΔ(→F1)+MΔ(→F2)+MΔ(→P)+MΔ(→P1)+MΔ(→P2)+MΔ(→R)=0
Ce qui donne : F1l1cosα−F2l2cosα+Mgacosα−mgl1sinα+mgl2sinα+0=0
Or, F1=F2=F donc, Fl1cosα−Fl2cosα+Mgacosα+mgsinα(l2−l1)=0
⇒ Fcosα(l1−l2)+Mgacosα+mgsinα(l2−l1)=0
⇒ F=Mgacosα+mgsinα×(l2−l1)(l2−l1)cosα
⇒ F=9.8300⋅10−3×6.0cos20∘+100⋅10−3×sin20∘×(24−12)(12+24)cos20∘
⇒F=0.61N
D'où, F=0.61N
3. Détermination du travail effectué par chaque force pendant deux tours θ=2π
Soit :
W(→F)=MΔ(→F)θ=F(l1+l2)×2π=0.61(12+24)10−2×2π=1.38
Donc, W(→F)=1.38J
On a :
W(→P)=MΔ(→P)θ=Mgacosα×2π=300⋅10−2×9.8×6.0⋅10−2cos20∘×2π=1.04
Donc, W(→P)=1.04J
Soit :
W(→P1)=MΔ(→P1)θ=−100⋅10−3×9.8×12⋅10−2sin20∘×2π=0.25
Ainsi, W(→P1)=0.25J
On a :
W(→P2)=MΔ(→P2)θ=100⋅10−3×9.8×24⋅10−2sin20∘×2π=0.51
Donc, W(→P2)=0.51J
Soit :
W(→R)=MΔ(→R)θ=0×2π=0
D'où, W(→R)=0J
Exercice 7
Un solide ponctuel S, de masse m, se déplace dans un plan vertical le long d'un trajet ABCD qui comporte deux phases.
− Une partie horizontale AB rectiligne de longueur 8m.
Le long de cette partie, le solide est soumis à une force constante →F, faisant un angle α=60∘ avec l'horizontale et développant une puissance P=6W en plus d'une force de frottement →f, opposée au déplacement de valeur constante f=3N.
− Une demi sphère BCD, de centre O et de rayon R=0.5m où le solide est soumis uniquement à son poids →P.
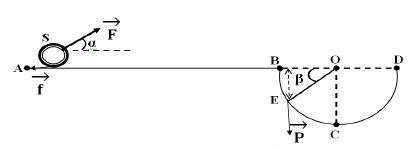
1. Sachant que pendant la partie AB le mouvement est rectiligne uniforme de vitesse V=2m⋅s−1,
a) Exprimons la puissance moyenne P développée par →F en fonction de F, V et α.
Soit :
Pm(→F)=→F⋅→V=FVcosα
b) Déduction de la valeur de la force F.
On a :
Pm(→F)=FVcosα⇒F=Pm(→F)Vcosα⇒F=62×cos60∘⇒F=6N
Donc, F=6N
c) Calcul du travail de la force →F pendant le déplacement AB.
Soit :
WAB(→F)=→F⋅→AB=F⋅ABcosα=6×8×cos60∘=24
D'où, WAB(→F)=24J
2. Détermination du travail de la force de frottement →f au cours du déplacement de AB.
On a :
WAB(→f)=→f⋅→AB=−f⋅ABcosα=−3×8=−24
Ainsi, WAB(→f)=−24J
3. Arrivant au point B, on annule les forces →F et →f.
Sachant que le travail du poids de S lorsqu'il glisse de B vers C est WB→C(→P)=0.5J
a) Déterminons la masse du solide S.
On a :
WB→C(→P)=mgR⇒m=WB→C(→P)gR⇒m=0.510×0.5⇒m=0.1kg
D'où, m=0.1kg
b) Donnons l'expression du travail du poids de S lorsqu'il passe de B vers E en fonction de m, g, R et β.
Soit : WB→E(→P)=mgRsinβ
Calcul de sa valeur : WB→E(→P)=0.1×10×0.5×sin30∘=0.25
Ainsi, WB→E(→P)=0.25J
c) Déduisons du travail du poids de S lors du déplacement de E vers C.
Soit :
WE→C(→P)=WB→C(→P)−WB→E(→P)=mgR−mgRsinβ=0.1×10×0.5(1−sin30∘)=0.25
Alors, WE→C(→P)=0.25J
4. Détermination du travail du poids de S lors du déplacement de C vers D.
Soit : WC→D(→P)=−WB→C(→P)
Donc, WC→D(→P)=−0.5J
Exercice 8
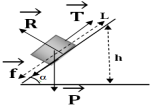
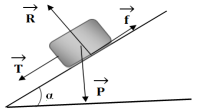
a. Bilan des forces extérieurs s'appliquant au traîneau
→T : force des traction exercée par les chiens,
→R : réaction du plan inclinée,
→f : force de frottement
et →P : Poids du traîneau
Représentation des forces sur un schéma

b. Résultante de ces forces
Le système évolue à vitesse constante, le principe de l'inertie s'écrit :
→T+→P+→R+→f=→O
La résultante de ces forces est donc nulle
c. Calcule du travail du poids →P et du travail de la force de frottement →f
W(→P)=−mgh=−mgLsinα=−110×500×6.0100⇒W(→P)=−33.103J
W(→f)=−70×500⇒W(→f)=−35⋅103J
d. Déduction du travail de la force de traction →T exercée par les chiens sur le traîneau pour un déplacement de longueur L
→T+→P+→R+→f=0⇒→T→L+→P→L+→R→L+→f→L=→O→L⇒W(T)+W(→P)+W(→R)+W(→f)=0
Or
W(→T)=0⇒W(→T)+W(→P)+W(→f)=⇒W(→T)=−W(→P)−W(→f)=−(−33⋅103)−(−35⋅103)⇒W(→T)=68⋅103J
Puissance moyenne de cette force
Pm(→T)=→T→V=TV or T=W(T)L⇒Pm(→T)=W(T)LV=68⋅103500×6.94⇒Pm(→T)=9.4⋅102W
Exercice 9


a. Calculer du travail du poids de l'échelle lors de cette opération.
W(→P)=−mgL2=−10×10×3.02⇒W(→P)=−1.5⋅102J
b. Calcul du travail du poids de l'échelle lors de cette opération.
W(→P)=−mgL2cosα=−10×10×3.02cos30∘⇒w(→P)=−1.3⋅102J
Exercice 10

a. Bilan des forces extérieures s'appliquant à la caravane et représentation de ces forces
→T : force de traction exercée par la voiture
→R : réaction du plan incliné
→f : Force de frottement
→P : poids de la caravane
b. Résultante de ces forces
Le système évolue à vitesse constante, le principe de l'inertie s'écrit :
→T+→P+→R+→f=→O
La résultante de ces forces est donc nulle
c. Calcul du travail du poids →P et du travail de la force de frottement →f pour un déplacement de longueur L
W(→P)=mgLsinα=500×10×200×6.0100=0⇒W(→P)=6⋅104J
or
W(→R)=0⇒W(→T)+W(→P)+W(→f)=0⇒W(→T)=−W(→P)−W(→f)=−6⋅104J−(−2.0⋅105J)⇒W(→R)=14⋅104J
W(→f)=−fT=−1.0⋅103×200⇒W(→f)=−2.0⋅105J
d. Déduction du travail de la force de la force de traction →T exercée par la voiture sur la caravane
W(→T)+W(→P)+W(→R)+W(→f)=0
La puissance moyenne de cette force
Pm(→T)=→T→V=TV or T=W(→T)L⇒Pm(→T)=W(→T)LV=14⋅104200×19.4⇒Pm(→T)=1.36⋅102W
e. La pente de la côte devrait être montante pour que le travail de →T change de signe
La signification physique de ce changement de signe permet de préciser la nature du travail de →T

Commentaires
Anonyme (non vérifié)
mer, 12/30/2020 - 23:22
Permalien
Bien
Abou (non vérifié)
mar, 10/12/2021 - 12:12
Permalien
Exercice 11 sur travail et puissance d'une force en 1ere
JEAN
sam, 01/06/2024 - 16:39
Permalien
Travail et puissance
Dione (non vérifié)
lun, 11/18/2024 - 17:58
Permalien
Correction des exercices
Ndiaye (non vérifié)
dim, 11/10/2024 - 17:21
Permalien
Merci beaucoup
amine (non vérifié)
lun, 01/04/2021 - 23:30
Permalien
Correction d’autres exercices
Dione (non vérifié)
mer, 01/20/2021 - 18:11
Permalien
Correction de l'exercice 11
Dozzh NGB (non vérifié)
mar, 11/09/2021 - 06:39
Permalien
Faire des Travaux dirigés
Dozzh NGB (non vérifié)
mar, 11/09/2021 - 06:39
Permalien
Faire des Travaux dirigés
Ngom (non vérifié)
mar, 11/07/2023 - 07:57
Permalien
Étude
Djafarou (non vérifié)
sam, 02/06/2021 - 22:18
Permalien
Physique
Anonyme (non vérifié)
dim, 01/22/2023 - 16:12
Permalien
C'est très bien votre site
Aly diop (non vérifié)
dim, 11/14/2021 - 23:34
Permalien
J’aimerais avoir le reste de
Fabiana (non vérifié)
mar, 11/19/2024 - 18:34
Permalien
Étude
Birahim fall (non vérifié)
jeu, 01/07/2021 - 07:19
Permalien
Regarder les autres corrections
Anonyme (non vérifié)
mar, 01/12/2021 - 22:55
Permalien
le probleme est que ont nous
Cheikh Diagne (non vérifié)
mer, 01/13/2021 - 13:39
Permalien
reussite
Elhadji (non vérifié)
mer, 11/01/2023 - 21:48
Permalien
Il m'a permis de mieux
Abdourahmane (non vérifié)
ven, 01/15/2021 - 01:10
Permalien
Eleve en première s2
ibrahima seck (non vérifié)
mer, 01/20/2021 - 20:55
Permalien
demande d'aide sur la science
Djafarou (non vérifié)
sam, 02/06/2021 - 22:20
Permalien
physique
Anonyme (non vérifié)
ven, 09/17/2021 - 16:01
Permalien
Ok
Djariatougassama (non vérifié)
ven, 10/29/2021 - 19:39
Permalien
Suivre les cours
Anonyme (non vérifié)
lun, 11/01/2021 - 18:43
Permalien
Salut
Divine (non vérifié)
lun, 11/01/2021 - 18:45
Permalien
Poids
Anonyme (non vérifié)
lun, 11/01/2021 - 18:47
Permalien
Salut
Anonyme (non vérifié)
mer, 09/21/2022 - 18:24
Permalien
Etude
Anonyme (non vérifié)
jeu, 09/22/2022 - 22:02
Permalien
La correction de l exo 5 me
Anonyme (non vérifié)
dim, 08/25/2024 - 23:56
Permalien
la correction de l exo 5
Anonyme (non vérifié)
dim, 08/25/2024 - 23:58
Permalien
La correction de l exo 5
Inass elfarouki (non vérifié)
dim, 09/25/2022 - 10:10
Permalien
Exercice corrige
Anonyme (non vérifié)
mar, 09/27/2022 - 18:22
Permalien
intéressant
Khady Ndiaye (non vérifié)
mar, 11/15/2022 - 21:37
Permalien
Sciences physiques
Hicham (non vérifié)
ven, 12/09/2022 - 19:27
Permalien
Ex:9
Anonyme (non vérifié)
mer, 11/01/2023 - 21:44
Permalien
C'est bonne
Anonyme (non vérifié)
mer, 11/01/2023 - 21:44
Permalien
C'est bonne
Anonyme (non vérifié)
mar, 08/01/2023 - 15:38
Permalien
Bonjour
Fatou seck (non vérifié)
dim, 11/12/2023 - 23:28
Permalien
Jeveux la c
KENE (non vérifié)
lun, 08/26/2024 - 00:02
Permalien
CORRECTION DE L EXO 5
Ajouter un commentaire