Corrigé devoir n° 3 maths - 2nd s

Classe: 
Seconde
 

Exercice 1

Soit ABC un triangle quelconque.
 
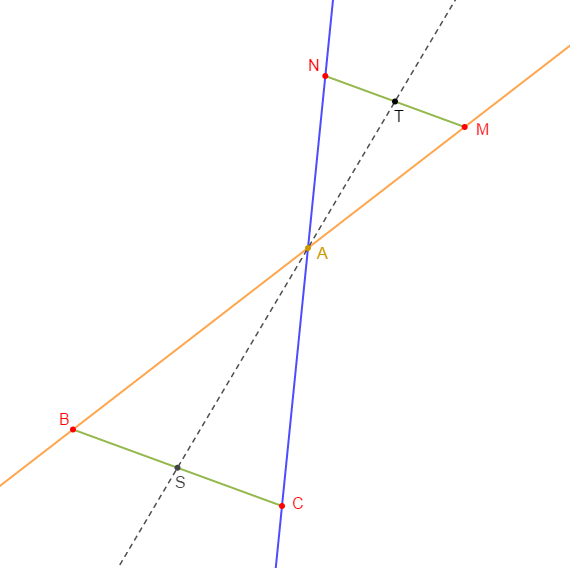
1) Construisons les points M  et  N tels que :
 
→AM=−23→AB  et  →AN=−23→AC
 
2) Démontrons que (MN)  et  (BC) sont parallèles.
 
D'une part, M; A; B sont trois points alignés dans cet ordre et d'autre part, N; A; C sont trois points alignés dans cet ordre.
 
De plus, ‖→AM‖‖→AB‖=23  et  ‖→AN‖‖→AC‖=23
 
Par ailleurs, on a :
 
→AN−→AM=→MN=−23→AC+23→AB=−23(→AC−→AB)=−23(→AC+→BA)=−23→BC
 
Alors, →MN=−23→BC
 
Par suite, ‖→MN‖‖→BC‖=23
 
Ainsi,
‖→AM‖‖→AB‖=‖→AN‖‖→AC‖=‖→MN‖‖→BC‖
Par conséquent, d'après la réciproque du théorème de Thalès, les droites (MN)  et  (BC) sont parallèles.
 
3) Soient S  et  T les milieux respectifs de [BC]  et  [MN]. Démontrons que les points A, S  et  T sont alignés.
 
Pour cela, il suffit de montrer que les vecteurs →AS et →AT sont colinéaires.
 
On a : →AM+→AN=−23→AB−23→AC
 
Alors, en appliquant la relation de Chasles, on obtient :
→AT+→TM+→AT+→TN=−23(→AS+→SB)−23(→AS+→SC)
Ce qui donne : 2→AT+→TM+→TN=−43→AS−23(→SB+→SC)
 
Or, S  et  T milieux respectifs de [BC]  et  [MN] donc,
 
→TM+→TN=→0  et  →SB+→SC=→0
 
Par suite, 2→AS=−43→AT
 
D'où, →AS=−23→AT
 
Ce qui prouve que les points A, S  et  T sont alignés.
 
 

Exercice 2

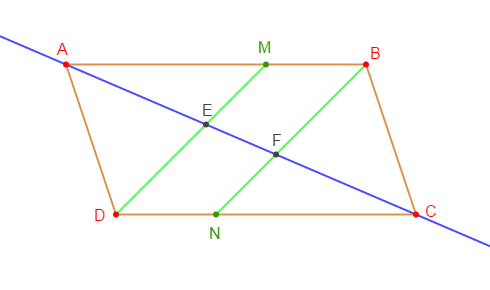
On considère un parallélogramme ABCD. Les points M et N sont tels que :
 
→AM=23→AB  et  →CN=23→CD
 
1) Faisons une figure.
 
2) Montrons que BMDN est un parallélogramme.
 
On a :
 
→AM+→CN=23→AB+23→CD=23(→AB+→CD)
 
Or, ABCD parallélogramme donc, →AB=−→CD ⇒ →AB+→CD=→0
 
Par suite,
 
→AM+→CN=→0⇒→AB+→BM+→CD+→DN=→0⇒→AB+→CD⏟=→0+→BM+→DN=→0⇒→BM+→DN=→0⇒→BM=→ND
 
Ainsi, →BM=→ND
 
Ce qui montre que BMDN est un parallélogramme.
 
3) Soit E le point commun aux droites (DM)  et  (AC).
 
Soit F le point commun aux droites (BN)  et  (AC).
 
Déterminons les réels a  et  b tels que :
→AE=a→AC  et  →AF=b→AC
A; E; F; C sont quatre points alignés dans cet ordre.
 
D'après la relation de Chasles, on a :
→AE+→EF+→FC=→AC
Or, les triangles AFB  et  AEM étant en position de Thalès alors, d'après le théorème de Thalès, on a :
 
‖→AF‖‖→AE‖=‖→AB‖‖→AM‖⇒‖→AE‖+‖→EF‖‖→AE‖=32⇒1+‖→EF‖‖→AE‖=32⇒‖→EF‖‖→AE‖=32−1⇒‖→EF‖‖→AE‖=12⇒‖→EF‖=12‖→AE‖
 
D'où, →EF=12→AE
 
De la même manière, en considérant les triangles CED  et  CFN qui sont en position de Thalès, on obtient :
 
‖→EC‖‖→FC‖=‖→DC‖‖→NC‖⇒‖→EF‖+‖→FC‖‖→FC‖=32⇒‖→EF‖‖→FC‖+1=32⇒‖→EF‖‖→FC‖=32−1⇒‖→EF‖‖→FC‖=12⇒‖→EF‖=12‖→FC‖
 
Donc, →EF=12→FC
{→EF=12→AE→EF=12→FC ⇒ →AE=→FC
Ainsi,
 
→AE+→EF+→FC=→AC⇒→AE+12→AE+→AE=→AC⇒(1+12+1)→AE=→AC⇒52→AE=→AC⇒→AE=25→AC
 
D'où, →AE=25→AC
 
Par ailleurs, d'après Thalès, on a : →AE=23→AF
 
Donc,
 
→AF=32→AE=32×25→AC=35→AC
 
D'où, →AF=35→AC
 
On peut aussi utiliser la relation de Chasles :
 
→AF=→AE+→EF=→AE+12→AE=32→AE=32×25→AC=35→AC
 
Ainsi, →AF=35→AC
 
 

Exercice 3

Soit ABC un triangle et un nombre x. A chaque valeur de x, on associe les points E et F tels que :
 
→AE=13→AB+x→AC  et  →AF=x→AB+13→AC
 
1) Faisons une figure lorsque x=−12.
 
 
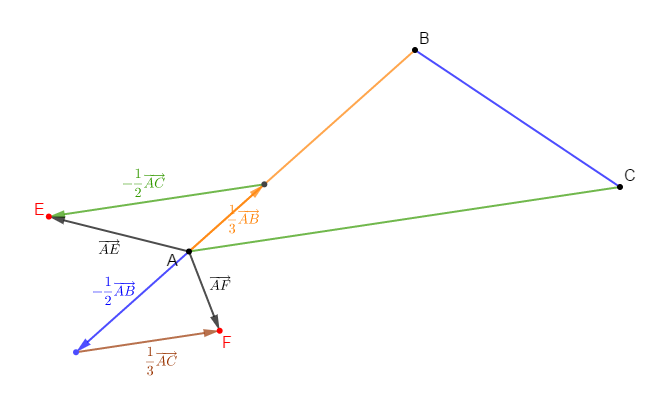
En effet, pour x=−12, on a :
→AE=13→AB−12→AC  et  →AF=−12→AB+13→AC
Donc, en considérant le repère (A; →AB; →AC), on place les points E  et  F.
 
2) Démontrons que, quel que soit le nombre x, les vecteurs →EF  et  →BC sont colinéaires.
 
En effet, d'après la relation de Chasles, on a :
 
→EF=→EA+→AF=−13→AB−x→AC+x→AB+13→AC=13(→AC−→AB)+x(→AB−→AC)=13(→AC+→BA)+x(→AB+→CA)=13→BC+x→CB=13→BC−x→BC=(13−x)→BC
 
D'où, →EF=(13−x)→BC
 
Ce qui prouve que, quel que soit le nombre x, les vecteurs →EF  et  →BC sont colinéaires.
 
3) Déterminons les valeurs de x pour que :
 
⋅ E  et  F confondus
 
E  et  F  sont confondus⇔→EF=→0⇔(13−x)→BC=→0or,  →BC≠→0⇔13−x=0⇔x=13
 
Donc, E  et  F sont confondus lorsque x=13
 
⋅ BCFE un parallélogramme
 
BCFE  est un parallélogramme⇔→EF=→BC⇔(13−x)→BC=→BC⇔(13−x)→BC−→BC=→0⇔(13−x−1)→BC=→0or,  →BC≠→0⇔13−x−1=0⇔x=13−1⇔x=−23
 
D'où, BCFE est un parallélogramme lorsque x=−23

Exercice 4

Résolvons dans R les équations et inéquations suivantes :
 
a) |7−5x|>3
 
|7−5x|>3⇔7−5x>3  ou  7−5x<−3⇔−5x>3−7  ou  −5x<−3−7⇔−5x>−4  ou  −5x<−10⇔x<45  ou  x>105=2⇔x∈]−∞; 45[∪]2; +∞[
 
Ainsi, S=]−∞; 45[∪]2; +∞[
 
b) |2x−3|≤52
 
|2x−3|≤52⇔−52≤2x−3≤52⇔−52+3≤2x≤52+3⇔12≤2x≤112⇔12×12≤x≤112×12⇔14≤x≤114⇔x∈[14; 114]
 
D'où, S=[14; 114]
 
c) |3−x2|=x3+6
 
|3−x2|=x3+6⇔3−x2=x3+6  ou  3−x2=−x3−6⇔−x2−x3=6−3  ou  −x2+x3=−6−3⇔−5x6=3  ou  −x6=−9⇔−5x=18  ou  −x=−54⇔x=−185  ou  x=54⇔x∈{−185; 54}
 
Ainsi, S={−185; 54}
 
d) |x−3|≤−2x+1
 
−  si −2x+1<0 alors, l'inéquation n'admet pas de solutions et donc, S=∅
 
On a :
 
−2x+1<0⇔−2x<−1⇔x>12
 
Ainsi, lorsque x∈]12; +∞[, S=∅
 
−  si −2x+1>0 alors, x∈]−∞; 12[
 
On a :
 
|x−3|≤−2x+1⇔{x−3≤−2x+12x−1≤x−3⇔{x+2x≤1+32x−x≤−3+1⇔{3x≤4x≤−2⇔{x≤43x≤−2
 
Donc, x∈]−∞; 12[∩]−∞; 43[∩]−∞; −2[
 
D'où, S=]−∞; −2[

Exercice 5(*)

1) a) Vérifions que pour tous réels strictement positifs a  et  x tels que : a>x, on a l'égalité :
 
x√a+x−√a−x=√a+x+√a−x2
 
En rendant rationnel le dénominateur du premier membre de cette égalité, on obtient :
 
x√a+x−√a−x=x(√a+x+√a−x)(√a+x−√a−x)(√a+x+√a−x)=x(√a+x+√a−x)(√a+x)2−(√a−x)2=x(√a+x+√a−x)a+x−a+x=x(√a+x+√a−x)2x=√a+x+√a−x2
 
Ainsi, x√a+x−√a−x=√a+x+√a−x2
 
b) En déduisons que pour tous réels strictement positifs a, m  et  p tels que : a>m>p, on a :
 
m√a+m−√a−m<p√a+p−√a−p
 
En appliquant les résultats de la question a) à chaque membre de l'inégalité, on obtient :
 
m√a+m−√a−m=√a+m+√a−m2
 
p√a+p−√a−p=√a+p+√a−p2
 
Donc,
 
m√a+m−√a−m<p√a+p−√a−p⇔√a+m+√a−m2<√a+p+√a−p2⇔(√a+m+√a−m2)2<(√a+p+√a−p2)2⇔14(√a+m+√a−m)2<14(√a+p+√a−p)2⇔(√a+m+√a−m)2<(√a+p+√a−p)2
 
On a :
 
(√a+m+√a−m)2=(√a+m)2+(√a−m)2+2√a+m√a−m=a+m+a−m+2√(a+m)(a−m)=2a+2√a2−m2
 
Aussi,
 
(√a+p+√a−p)2=(√a+p)2+(√a−p)2+2√a+p√a−p=a+p+a−p+2√(a+p)(a−p)=2a+2√a2−p2
 
Ainsi, en faisant la différence de ces deux termes, on obtient :
 
(√a+m+√a−m)2−(√a+p+√a−p)2=2a+2√a2−m2−2a−2√a2−p2=2(√a2−m2−√a2−p2)
 
Par ailleurs, on a :
 
(√a2−m2)2=a2−m2
 
(√a2−p2)2=a2−p2
 
Par suite, la différence de ces termes donne :
 
(√a2−m2)2−(√a2−p2)2=a2−m2−a2+p2=−m2+p2=−(m2−p2)=−(m−p)(m+p)<0
 
D'où, √a2−m2−√a2−p2<0
 
Par conséquent,
 
\dfrac{m}{\sqrt{a+m}-\sqrt{a-m}}<\dfrac{p}{\sqrt{a+p}-\sqrt{a-p}}
 
2) Simplifions l'expression :
\dfrac{1}{(a+b)^{2}}\left(\dfrac{1}{a^{2}}+\dfrac{1}{b^{2}}\right)+\dfrac{2}{(a+b)^{3}}\left(\dfrac{1}{a}+\dfrac{1}{b}\right)
Soit :
 
\begin{array}{rcl}\dfrac{1}{(a+b)^{2}}\left(\dfrac{1}{a^{2}}+\dfrac{1}{b^{2}}\right)+\dfrac{2}{(a+b)^{3}}\left(\dfrac{1}{a}+\dfrac{1}{b}\right)&=&\dfrac{a^{2}+b^{2}}{(a+b)^{2}a^{2}b^{2}}+\dfrac{2(a+b)}{ab(a+b)^{3}}\\\\&=&\dfrac{1}{ab(a+b)^{2}}\left(\dfrac{a^{2}+b^{2}}{ab}+\dfrac{2(a+b)}{(a+b)}\right)\\\\&=&\dfrac{1}{ab(a+b)^{2}}\left(\dfrac{a^{2}+b^{2}}{ab}+2\right)\\\\&=&\dfrac{1}{ab(a+b)^{2}}\left(\dfrac{a^{2}+b^{2}+2ab}{ab}\right)\\\\&=&\dfrac{1}{ab(a+b)^{2}}\left(\dfrac{(a+b)^{2}}{ab}\right)\\\\&=&\dfrac{(a+b)^{2}}{a^{2}b^{2}(a+b)^{2}}\\\\&=&\dfrac{1}{a^{2}b^{2}}\end{array}
 
D'où, \boxed{\dfrac{1}{(a+b)^{2}}\left(\dfrac{1}{a^{2}}+\dfrac{1}{b^{2}}\right)+\dfrac{2}{(a+b)^{3}}\left(\dfrac{1}{a}+\dfrac{1}{b}\right)=\dfrac{1}{(ab)^{2}}}

 

 
Auteur: 
Diny Faye

Commentaires

ousmane sankhare (non vérifié)

lun, 11/01/2021 - 08:52

Démonstration très riche

Anonyme (non vérifié)

mer, 12/20/2023 - 22:45

trés intréssant

Ajouter un commentaire